Triangle area calculator

Understanding the area of a triangle

The area of a triangle is a fundamental concept in geometry that represents the total space enclosed by its three sides. Understanding this concept is crucial because it allows us to quantify the size of triangular shapes, which are prevalent in various fields such as architecture, engineering, and art.

The area of a triangle can vary significantly depending on its type-whether it's scalene, isosceles, or equilateral. Each type has unique properties that affect how we calculate its area.

When discussing the area of triangles, it's essential to mention the units used to express this measurement. Typically, the area is expressed in square units like square meters (m²), square centimeters (cm²), or square inches (in²). These units help standardize measurements and make it easier to compare different triangles. Calculating the area of a triangle involves understanding these variations and applying appropriate formulas based on the given dimensions. Whether you're dealing with a right-angled triangle or an obtuse one, knowing how to compute its area accurately is invaluable.

Here are some key points to consider:

• Scalene Triangle: All sides have different lengths, requiring specific calculations.
• Isosceles Triangle: Two sides are equal, simplifying some calculations.
• Equilateral Triangle: All sides and angles are equal, allowing for straightforward area computation.

Basic formula for calculating triangle area

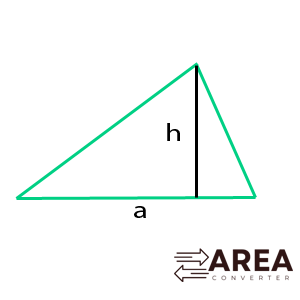
The basic formula for calculating the area of a triangle is straightforward and essential for anyone dealing with geometric shapes. This formula, A = 1/2 × base × height, allows you to determine the space enclosed by a triangle in a two-dimensional plane. The base refers to any side of the triangle, while the height is the perpendicular distance from the base to the opposite vertex. This method is applicable to all triangles, whether they are scalene, isosceles, or equilateral.

• Example 1: If you have a triangle with a base of 10 inches and a height of 5 inches, the area would be calculated as follows: A = 1/2 × 10 × 5 = 25 square inches.
• Example 2: Consider a triangle with a base of 8 cm and a height of 7 cm. Using the formula, the area would be A = 1/2 × 8 × 7 = 28 square centimeters.

These examples illustrate how simple it can be to apply this formula once you know the base and height. By mastering this basic calculation, you'll be well-equipped to tackle more advanced problems involving triangles in various fields such as architecture and engineering.

Using Heron's formula for triangle area

Heron's formula is a powerful tool for calculating the area of a triangle when you know the lengths of all three sides. This method is particularly useful when the height of the triangle is not readily available, making it an essential technique in various mathematical and practical applications. To apply Heron's formula, you first need to determine the semi-perimeter of the triangle, which is half the sum of its three sides. The formula itself is expressed as:

• Step 1: Calculate the semi-perimeter (s) using the formula: \( s = \frac{a + b + c}{2} \), where \( a \), \( b \), and \( c \) are the lengths of the sides.
• Step 2: Use Heron's formula to find the area: \( \text{Area} = \sqrt{s(s-a)(s-b)(s-c)} \).

Let's consider an example to illustrate how Heron's formula works in practice. Suppose you have a triangle with side lengths of 7 cm, 8 cm, and 9 cm. First, calculate the semi-perimeter: \( s = \frac{7 + 8 + 9}{2} = 12 \). Then, substitute these values into Heron's formula to find the area:

• \( \text{Area} = \sqrt{12(12-7)(12-8)(12-9)} \)
• \( \text{Area} = \sqrt{12 \times 5 \times 4 \times 3} = \sqrt{720} = 26.83\) square centimeters.

This step-by-step approach ensures accuracy and provides a clear understanding of how Heron's formula can be applied effectively. Whether you're working on a geometry problem or tackling real-world challenges in fields like architecture or engineering, mastering this technique can be incredibly beneficial.

Calculating area with two Sides and an included angle

When you need to calculate the area of a triangle and you know two sides along with the included angle, there's a straightforward method to follow. This approach is particularly useful in scenarios where traditional base-height measurements are not available. The formula used in this case is derived from trigonometry and is expressed as: Area = 1/2 × side1 × side2 × sin(angle). Here, the angle is the one formed between the two known sides. This formula leverages the sine function to account for the angle's influence on the area, making it a versatile tool in geometry.

To illustrate this method, consider a triangle where side a measures 7 units, side b measures 9 units, and the included angle C is 30 degrees. Using our formula, we calculate: Area = 1/2 × 7 × 9 × sin(30°). Since sin(30°) equals 0.5, the area becomes 15.75 square units. This technique is not only efficient but also essential for various applications such as architecture and engineering, where precise measurements are crucial. Remember these key points when applying this method:

• The angle must be between the two known sides.
• The sine function is critical for accuracy.
• This formula adapts well to different triangle configurations.

Special cases: Right, equilateral, and isosceles triangles

When it comes to calculating the area of triangles, special cases like right-angled, equilateral, and isosceles triangles have their own unique formulas. Understanding these can simplify your calculations significantly. For a right-angled triangle, the formula is straightforward: the area is half the product of its base and height. This is because one of the sides acts as the height, making it easy to apply the basic formula. In contrast, an equilateral triangle, where all sides are equal, uses a different approach. The area is calculated using the formula: \(A = \frac{\sqrt{3}}{4} \times \text{side}^2\). This formula leverages the symmetry of equilateral triangles to provide a quick solution.

On the other hand, an isosceles triangle, which has two equal sides, requires another specific method. The area can be found using: \(A = \frac{1}{4}b\sqrt{4a^2 - b^2}\), where 'b' is the base and 'a' is one of the equal sides. Each type of triangle offers a unique way to calculate its area, reflecting its geometric properties. Here’s a quick summary:

By understanding these formulas, you can efficiently tackle problems involving these specific types of triangles in various practical scenarios.