Area of an ellipse calculator

The ellipse area calculator is a handy tool for quickly finding the area of an ellipse. In this guide, you'll explore how the calculator works and gain insights into the concept of an ellipse, including its area formula.

Dive deeper to uncover the definition of an ellipse, learn about its foci, and explore the standard ellipse equation. For those already familiar with the basics and seeking a more detailed analysis, consider trying our advanced ellipse calculator for more complex computations.

Ellipse calculator

Semi-major axis (x)

Semi-major axis (y)

Area

Definition: What is an ellipse?

An ellipse is a fundamental geometric shape that appears in various fields, from mathematics and physics to engineering and astronomy. Formally, an ellipse is defined as the set of all points in a plane such that the sum of their distances from two fixed points, called the foci, is constant. This unique property distinguishes the ellipse from other conic sections such as circles, parabolas, and hyperbolas.

Mathematically, an ellipse can be represented by its standard equation:

x²/a² + y²/b² = 1

Here:

a is the length of the semi-major axis (the longer radius),
b is the length of the semi-minor axis (the shorter radius),
and the center of the ellipse is at the origin (0,0) in a Cartesian coordinate system.

Understanding the components of an ellipse

Major axis and minor axis: The longest and shortest diameters of the ellipse, respectively. The major axis passes through both foci, while the minor axis is perpendicular to it.

Foci (singular: focus): These are two distinct points inside the ellipse. The constant sum of distances to the foci defines the shape.

Center: The midpoint of both the major and minor axes, serving as the symmetry point of the ellipse.

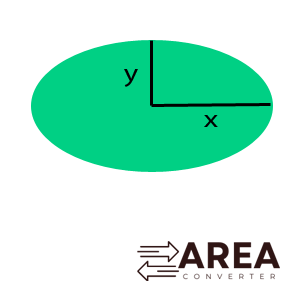
How to calculate the area of an ellipse?

Calculating the area of an ellipse is straightforward when you know the lengths of its semi-major axis (a) and semi-minor axis (b). The formula for the area of an ellipse is derived from its geometric properties:

Area = π x a x b

Here:

a is the length of the semi-major axis (half the longest diameter),
b is the length of the semi-minor axis (half the shortest diameter),

Step-by-Step calculation

Measure the axes

Determine the length of the semi-major axis (a).
Determine the length of the semi-minor axis (b).

Apply the formula

Multiply π by the product of a and b.

Compute the area

Use a calculator to perform the multiplication for precise results.